Assessment |
Biopsychology |
Comparative |
Cognitive |
Developmental |
Language |
Individual differences |
Personality |
Philosophy |
Social |
Methods |
Statistics |
Clinical |
Educational |
Industrial |
Professional items |
World psychology |
Biological: Behavioural genetics · Evolutionary psychology · Neuroanatomy · Neurochemistry · Neuroendocrinology · Neuroscience · Psychoneuroimmunology · Physiological Psychology · Psychopharmacology (Index, Outline)

Current flowing through a wire produces a magnetic field (B, labeled M here) around the wire. The field is oriented according to the right-hand rule.
- For other senses of this term, see magnetic field (disambiguation).
.
In physics, a magnetic field is relativistic part of electric field (as explained by Einstein in 1905). When electric charge is moving by observer, the electric field of this charge due to space contraction no longer seen by the observer as spherically symmetric (nor even as radial) but must be computed using Lorentz transformations. One of products of these transformations is the part of electric field which only acts on moving charges - and we call it "magnetic field".
The quantum-mechanical motion of electrons in atoms produces magnetic fields of permanent ferromagnets. Spinning charged particles also have magnetic moment. Some electrically neutral particles (like neutron) with non-zero spin also have magnetic moment due to charge distribution in their inner structure. Spin-zero particles never have magnetic moment.
A magnetic field is a vector field: it associates with every point in space a (pseudo-)vector that may vary in time. The direction of the field is the equilibrium direction of a compass needle (=magnetic dipole) placed in the field.
Symbols and terminology[]
Magnetic field is usually denoted by the symbol . Historically, was called the magnetic flux density or magnetic induction. A distinct quantity, , was called the magnetic field, and this terminology is still often used to distinguish the two in the context of magnetic materials (non-trivial permeability μ). Otherwise, however, this distinction is often ignored, and both quantities are frequently referred to as "the magnetic field." (Some authors call H the auxiliary field, instead.) In linear materials, such as air or free space, the two quantities are linearly related:
where is the magnetic permeability (in henries per meter) of the medium.
In SI units, and are measured in teslas (T) and amperes per meter (A/m), respectively; or, in cgs units, in gauss (G) and oersteds (Oe), respectively. Two parallel wires carrying an electric current in the same sense will generate a magnetic field which will cause a force of attraction to each other. This fact is used to generate the value of an ampere of electric current. Note that while like charges repel and unlike ones attract, the opposite holds for currents: if the current in one of the two parallel wires is reversed, the two will repel.
Definition[]
Lorentz transformation of spherically symmetric proper electric field E of moving electric charge (for example, electric field of an electron moving in a conducting wire) from charge's reference frame to non-moving observer's reference frame results in the following term:
which we label as "magnetic field" and use the symbol B for it for the sake of mathematical simplicity.
As seen from the definition, the unit of magnetic field is newton-second per coulomb-meter (or newton per ampere-meter) and is called the tesla.
Like the electric field, the magnetic field exerts force on electric charge—but only on moving charge:
where
- F is the force produced, measured in newtons
- indicates a vector cross product
- is electric charge that the magnetic field is acting on, measured in coulombs
- is velocity of the electric charge , measured in metres per second
Because magnetic field is the relativistic product of Lorentz transformations, the force it produces is called the Lorentz force.
The force due to the magnetic field is different in different frames—moving magnetic field transforms partially or fully back into electric fields under Lorentz transformations. This results in Faraday induction law.
Magnetic field of flow (current) of charged particles[]
Substituting into the definition of magnetic field
the proper electric field of point-like charge (see Coulomb's law)
results in the equation:
This equation is usually called Biot-Savart law.
Here : is electric charge motion of which creates the magnetic field, measured in coulombs
- is velocity of the electric charge that is generating B, measured in metres per second
- B is the magnetic field (measured in teslas)
Lorentz force on wire segment[]
The Lorentz force on a stationary wire carrying moving charges (=current) is therefore:
where
- F = force (newton)
- B = flux density (tesla)
- L = length of wire (metre)
- i = current in wire (ampere)
In the equation above, the current vector i is a vector with magnitude equal to the scalar current, i, and direction pointing along the wire that the current is flowing. Alternatively, instead of current the wire segment can be considered a vector.
Vector calculus[]
Separating electric field of moving charge into stationary electric and stationary magnetic components (= as measured by stationary observer)—which are usually labeled as E and B—replaces complex Einstein relativistic field transformation equations by more compact and elegant mathematical statements known as Maxwell equations. Two of them which describe magnetic component are:
where
- is the curl operator
- is the divergence operator
- is permeability
- is current density
- is the partial derivative
- is the free-space permittivity
- is the electric field
- is time
The first equation is known as Ampère's law with James Clerk Maxwell's correction. The second term of this equation (Maxwell's correction) disappears in static (time independent) systems. The second equation is a statement of the observed non-existence of magnetic monopoles. These are two of four Maxwell's equations written in differential notation (introduced by Oliver Heaviside).
Energy in the magnetic field[]
If to divide the energy of a long (or toroidal) solenoid by the volume of the solenoid, the density of magnetic field energy can be obtained:
For example, magnetic field B = 1 tesla has energy density about 398 kilojoules per cubic meter, and of 10 teslas, about 40 megajoules per cubic meter.
The same is the pressure produced by magnetic field (pressure and energy density are essentially the same physical quantities and thus have the same units). Thus, magnetic field of 1 tesla produces pressure of 398 kPa (about 4 atmospheres), and 10 T about 40 Mpa (~400 atm).
Properties[]
Maxwell did much to unify static electricity and magnetism, producing a set of four equations relating the two fields. However, under Maxwell's formulation, there were still two distinct fields describing different phenomena. It was Albert Einstein who showed, using special relativity, that electric and magnetic fields are two aspects of the same thing (a rank-2 tensor), and that one observer may perceive a magnetic force where a moving observer perceives only an electrostatic force. Thus, using special relativity, magnetic forces are a manifestation of electrostatic forces of charges in motion and may be predicted from knowledge of the electrostatic forces and the movement (relative to some observer) of the charges.
Changing magnetic field is mathematically the same as moving magnetic field (see relativity of motion)—thus according to Einstein's field transformation equations (= Lorentz transformation of field from proper reference frame to non-moving reference frame) part of it appears as electric field component—this is known as Faraday's law of induction and is the principle behind electric generators and electric motors.
Magnetic field lines[]
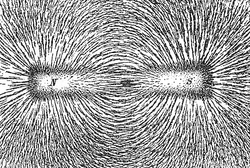
The direction of magnetic field lines is defined as the direction of orientation of magnetic dipole—say, a small magnet or a loop of current in the magnetic field.
Pole labeling confusions[]
The "north" and "south" poles of a magnet or a magnetic dipole are labelled somewhat confusingly in comparison to the geographic north and south poles. In particular, the "north pole" of the Earth's magnetic dipole moment is located at the geographic (magnetic) south pole and vice versa.
By convention, the pole of a magnet is labelled according to the geographic direction it points in the presence of the Earth's magnetic field. Hence, when we speak of the "north pole" of a magnet, it is a reference to the "north-seeking" pole. Equivalently, magnetic field lines run from the north to the south pole of a magnet. (The geomagnetic field lines thus run from south to north along the Earth's surface.)
Rotating magnetic fields[]
A rotating magnetic field is a magnetic field which rotates in polarity at non-relativistic speeds. This is a key principle to the operation of alternating-current motor. A permanent magnet in such a field will rotate so as to maintain its alignment with the external field. This effect is utilised in alternating current electric motors. Rotating magnetic field can be constructed using three or more phase alternating currents. Synchronous motors and induction motors use a stator's rotating magnetic fields to turn rotors.
In 1882, Nikola Tesla identified the concept of the rotating magnetic field. In 1885, Galileo Ferraris independently researched the concept. In 1888, Tesla gained U.S. Patent 381,968
for his work. Also in 1888, Ferraris published his research in a paper to the Royal Academy of Sciences in Turin.
Hall effect[]
Because Lorentz force is charge sign dependent (see above), it results in a charges separation, when a conductor with curent is placed in transverse magnetic field—with a buildup of opposite charges on two opposite sides of conductor (in the direction normal to the magnetic field direction)—and the potential difference between these sides can be measured.
Hall effect is often used to measure the magnitude of magetic field.
See also[]
General
- Electric field - effect produced by an electric charge that exerts a force on charged objects in its vicinity.
- Electromagnetic field - a field composed of two related vector fields, the electric field and the magnetic field.
- Electromagnetism - the physics of the electromagnetic field: a field, encompassing all of space, composed of the electric field and the magnetic field.
- Magnetism - phenomenon by which materials exert an attractive or repulsive force on other materials.
- Magnetohydrodynamics - the academic discipline which studies the dynamics of electrically conducting fluids.
Mathematics
- Ampere's law - magnetic equivalent of Gauss's law.
- Biot-Savart law - describes the magnetic field set up by a steadily flowing line current.
- Magnetic helicity - extent to which a magnetic field "wraps around itself".
- Maxwell's equations - four equations describing the behavior of the electric and magnetic fields, and their interaction with matter.
Applications
- Helmholtz coil - a device for producing a region of nearly uniform magnetic field.
- Maxwell coil - a device for producing a large volume of almost constant magnetic field.
- Earth's magnetic field - a discussion of the magnetic field of the Earth.
- Dynamo theory - a proposed mechanism for the creation of the Earth's magnetic field.
- Electric motor - AC motors used magnetic fields
References[]
Books
- Griffiths, David J. (1998). Introduction to Electrodynamics (3rd ed.), Prentice Hall. ISBN 013805326X.
- Jackson, John D. (1998). Classical Electrodynamics (3rd ed.), Wiley. ISBN 047130932X.
- Tipler, Paul (2004). Physics for Scientists and Engineers: Electricity, Magnetism, Light, and Elementary Modern Physics (5th ed.), W. H. Freeman. ISBN 0716708108.
External articles[]
Information
- Nave, R., "Magnetic Field". HyperPhysics.
- "Magnetism", The Magnetic Field. theory.uwinnipeg.ca.
- Hoadley, Rick, "What do magnetic fields look like?" 17 July 2005.
Rotating magnetic fields
- "Rotating magnetic fields". Integrated Publishing.
- "Introduction to Generators and Motors", rotating magnetic field. Integrated Publishing.
- "Induction Motor-Rotating Fields".
Diagrams
- McCulloch, Malcolm,"A2: Electrical Power and Machines", Rotating magnetic field. eng.ox.ac.uk.
- "AC Motor Theory" Figure 2 Rotating Magnetic Field. Integrated Publishing.
Journal Articles
- Yaakov Kraftmakher, "Two experiments with rotating magnetic field". 2001 Eur. J. Phys. 22 477-482.
- Bogdan Mielnik and David J. Fernández C., "An electron trapped in a rotating magnetic field". Journal of Mathematical Physics, February 1989, Volume 30, Issue 2, pp. 537-549.
- Sonia Melle, Miguel A. Rubio and Gerald G. Fuller "Structure and dynamics of magnetorheological fluids in rotating magnetic fields". Phys. Rev. E 61, 4111–4117 (2000).
ar:حقل مغناطيسي da:Magnetfelt de:Magnetfeld es:Campo magnético fr:Champ magnétique he:שדה מגנטי ru:Магнитное поле sk:Magnetické pole sl:Magnetno polje th:สนามแม่เหล็ก vi:Từ trường
| This page uses Creative Commons Licensed content from Wikipedia (view authors). |

























