Assessment |
Biopsychology |
Comparative |
Cognitive |
Developmental |
Language |
Individual differences |
Personality |
Philosophy |
Social |
Methods |
Statistics |
Clinical |
Educational |
Industrial |
Professional items |
World psychology |
Biological: Behavioural genetics · Evolutionary psychology · Neuroanatomy · Neurochemistry · Neuroendocrinology · Neuroscience · Psychoneuroimmunology · Physiological Psychology · Psychopharmacology (Index, Outline)
Membrane potential (or transmembrane potential or transmembrane potential difference or transmembrane potential gradient), is the electrical potential difference (voltage) across a cell's plasma membrane. In membrane biophysics it is sometimes used interchangeably with cell potential, but is applicable to any lipid bilayer or membrane. Hence every organelle and every membranous compartment (such as a synthetic vesicle) has a transmembrane potential (although the size of this potential may be zero).
Described in physical terms, it is the voltage "drop" or the difference in potential between one face of a bilayer and its immediate opposite face. The property need not be uniform throughout the cell or compartment, but under some conditions may vary between one patch of membrane and another. A localized change in potential occurs at the synapse of nerve cell, for example, with the opening of ion channels by neurotransmitters. Likewise during an action potential, the magnitude of the membrane potential will vary in time and space along a nerve fiber. Action potentials are complex temporal and spatial patterns of membrane potential change. Action potentials are transient spikes in membrane potential characterized by initiating membrane potential changes that start the transmembrane flow of charged solutes through voltage-gated ion channels which in turn results in new membrane potential changes that ultimately return the membrane potential to the value it had at the start of the spike.
Neural cells fire an action potential when the membrane potential exceeds a threshold value, triggering the opening of voltage-gated ion channels. Establishing a membrane potential artificially allows such firing to be induced as well.
A graded membrane potential is a gradient of transmembrane potential difference along a length of cell membrane. Graded potentials are particularly important in neurons that lack action potentials, such as some types of retinal neurons. Graded potentials that depolarize the membrane, reducing the membrane potential below the resting potential are important as "triggering potentials" that can spread along the surface of neuronal cell bodies to axon initial segments and trigger action potentials. Graded potentials that hyperpolarize the membrane potential to values more negative than the resting potential can inhibit the generation of action potentials. Graded potentials can arise at either portions of cells that function as sensory receptors or at synapses that are activated by neurotransmitters. These two types of graded potentials are called receptor potentials or synaptic potentials. Graded potentials are distinct from action potentials in that graded potentials spread electric potential changes along cell membranes without activating the kind of constant magnitude propagating signal that is characteristic of the action potential. Graded potentials are highest at a source and decay with increasing distance from the source.
The Ionic Basis of the resting potential[]
At its core, the origin of every resting membrane potential is some sort of ion pump exchanger or transporter. Any voltage - membrane potentials included - is a separation of charges across a resistive barrier. The typical membrane potential of a cell arises from the separation of potassium ions from intracellular immobile anions across the membrane of the cell. In order for this separation to occur, a concentration gradient of potassium ions must first be set up. While most descriptions of the genesis of membrane potential begin with the concentration gradients already in place, as if by magic (conservation of energy be damned!), these gradients must be created by putting energy into the system. This work is done by the ion pumps/transporters and/or exchangers and generally is powered by ATP. In the case of the standard membrane potential across a cell's plasma membrane, potassium (and sodium) gradients are established by the NaKATPase (sodium potassium exchanger). In other cases for example, a membrane potential may be established by acidification of the inside of a membranous compartment (such as the proton pump that generates membrane potential across synaptic vesicle membranes.
In its most simple terms one can trace the origin of the energy of the membrane potential (voltage is, after all, potential energy) thus:
Solar energy → converted to sugar by plants → converted by glycolysis to ATP → converted to concentration gradient by NaKATPase (sodium/potassium pump) → converted to voltage by passive K+ transport across a selectively permeable cell membrane.
Generation of the resting potential[]
Cell membranes are typically permeable to a only a subset of ionic species. These species usually include potassium ions, chloride ions, bicarbonate ions, and others. To simplify the description of the ionic basis of the resting membrane potential, it is most useful to consider only one ionic species at first, and consider the others later. Since trans-plasma-membrane potentials are almost always determined primarily by potassium permeability, that is where to start.
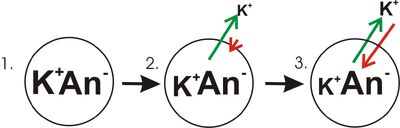
A diagram showing the progression in the development of a membrane potential from a concentration gradient (for potassium). Green arrows indicate net movement of K+ down a concentration gradient. Red arrows indicate net movement of K+ due to the membrane potential. The diagram is misleading in that while the concentration of potassium ions outside of the cell increases, only a small amount of K+ needs to cross the membrane in order to produce a membrane potential with a magnitude large enough to counter the tendency the potassium ions to move down the concentration gradient.
- Panel 1 of the diagram shows a digramatic representation of a simple cell where a concentration gradient has already been established. This panel is drawn as if the membrane has no permeability to any ion. There is no membrane potential, because despite there being a concentration gradient for potassium, there is no net charge imbalance acrosss the membrane. If the membrane were to become permeable to a type of ion that is more concentrated on one side of the membrane, then that ion would contribute to membrane voltage because the permeant ions would move across the membrane with net movement of that ion type down the concentration gradient. There would be net movement from the side of the membrane with a higher concentration of the ion to the side with lower concentration. Such a movement of one ion across the membrane would result in a net imbalance of charge across the membrane and a membrane potential. This is a common mechanism by which many cells establish a membrane potential.
- In panel 2 of the diagram, the cell membrane has been made permeable to potassium ions, but not the anions (An-) inside the cell. These anions are mostly contributed by protein. There is energy stored in the potassium ion concentration gradient that can be converted into an electrical gradient when potassium (K) ions move out of the cell. Note that K ions can move across the membrane in both directions but by the purely statistical process that arises from the higher concentration of K inside the cell, there will be more K ions moving out of the cell. Because there is a higher concentration of K ions inside the cells, their random molecular motion is more likely to encounter the permeability pore (ion channel) than is the case for the K ions that are outside and at a lower concentration. An internal K+ is simply "more likely" to leave the cell than an extracellular K+ is to enter it. It is a matter of simple diffusion doing work by dissipating the concentration gradient. As potassium leaves the cell, it is leaving behind the anions. Therefore a charge separation is developing as K+ leaves the cell. This charge separation creates a transmembrane voltage. This transmembrane voltage is the membrane potential. As potassium continues to leave the cell, separating more charges, the membrane potential will continue to grow. The length of the arrows (green indicating concentration gradient, red indicating voltage), represents the magnitude of potassium ion movement due to each form of energy. The direction of the arrow indicates the direction in which that particular force is applied. Thus, the building membrane voltage is an increasing force that acts counter to the tendency for net movement of K ions down the potassium concentration gradient.
- In Panel 3, the membrane voltage has grown to the extent that its "strength" now matches the concentration gradient's. Since these forces (which are applied to K+ions) are now the same strength and oriented in opposite directions, the system is now in equilibrium. Put another way, the tendency of potassium to leave the cell by running down its concentration gradient is now matched by the tendency of the membrane voltage to pull potassium ions back into the cell. K+ continues to move across the membrane, but the rate at which it enters and leaves the cell are the same, thus, there is no net potassium current. Because the K+ is at equilibrium, membrane potential is stable, or "resting".
The number of ions involved in generating the resting potential[]
You may have noticed that in the diagram above, while the red arrow (with length proportional to the voltage) was growing, the green arrow (with length proportional to the concentration gradient) was not changing significantly. How can this be? If K+ is leaving the cell, won't this deplete the cell of intracellular K+, thus decreasing the concentration gradient? The answer to the latter question is "not much", the concentration gradient is not dissipated very much. So how can this be? To answer this question, one must understand is that the number of potassium ions that must cross the membrane is actually quite small. This is because the membrane potential occurs only right next to the membrane. The membrane potential is stored in the membrane capacitance, which physically, is only the charges in direct contact with the membrane surfaces. The voltage across the capacitor is the membrane potential (see action potential for an example of a membrane RC circuit). Knowing that, one needs only to calculate how much current needs to flow to discharge the membrane capacitance to calculate the number of charges that must flow across the membrane to change the capacitor voltage (membrane potential) by, say 100 mV.
The capacitative current that flows into or out of a capacitor to effect a change in membrane potential of amplitude dV, is given by the equation:
Ic = C dV/dt (capacitative current = capacitance times the voltage change over time).
To make a back-of-the-envelope calculation, let’s stipulate an instantaneous change in voltage of 100 mV (i.e. dt=0; this gets rid of the time variable and simplifies the equation to:
- Ic=C dV (capacitative current = capacitance times the change in voltage (basically a variation on Ohm's law where capacitance substitutes for resistance).
- The generally accepted value for membrane capacitance (C) is 1 microfarad per square centimeter.
- The voltage change dV is 0.1 volts.
- Plugging those numbers into the formula, yields a capacitative current of 100 nanoamperes (1×10 -7 amperes).
- So it takes 1×10 -7 amperes to move the membrane potential by 100 mV
- An ampere is 1 coulomb per second.
- Since we are doing an instantaneous calculation, lets just say that time equals one second so that 1×10 -7 amperes = 1×10 -7 coulombs.
- One coulomb is 6.25 X 1018 elementary charges.
- So it takes 1×10 -7 amperes to move the membrane potential by 100 mV
- Therefore, it would take the movement of 6.25 X 1011 charges across the membrane to change the voltage of 1 square centimeter of membrane by 100 mV.
- However, even the largest mammalian brain neurons have a surface area far smaller than 1 square centimeter.
- For example, the total surface area of a spherical cell 50 micrometers in diameter is only 8×10-9 square meter, or 8×10-5 square centimeter.
- Thus, the capacitance of such a cell is much smaller than it would be for a cell with a surface area of 1 cm2.
- For example, the total surface area of a spherical cell 50 micrometers in diameter is only 8×10-9 square meter, or 8×10-5 square centimeter.
- Correcting for the smaller surface area (and thus smaller C) moving the membrane potential by 100 mV would require only 6.25 X 104 charges.
- The bottom line for those who don’t want to sift through the calculation is that only about 60,000 potassium ions must cross the membrane to move the membrane potential by 100 mV.
- Another back of the envelope calculation shows that the inside of that 50 micrometer diameter contains approximately 36 billion potassium ions. This means that only 0.00001% of a cell's potassium ions must cross the membrane to generate a membrane potential of 100 mV. Therefore, dissipation of the membrane ion concentration gradient is negligible.
Equilibrium potentials[]
An equilibrium potential is the membrane voltage at which a particular ion is in equilibrium. The equilibrium potential (also called reversal potential or Nernst Potential) is the membrane voltage at which the voltage force exactly balances the concentration gradient force (see section above), thus the voltage at which the inward and outward flows of the ion are balanced (net current = zero), or in equilibrium. The equilibrium potential of a particular ion is designated by the notation Eion. In the previous section, the equilibrium potential specifically for potassium ions was described, but any permeant ion will have an equilibrium potential that is determined by the concentration gradient for that ion across the membrane. For potassium, EK is typically around −80 mV. For sodium, ENa is typically around +60 mV. Note that the sign of ENa and EK are opposite. This is because the concentration gradient for potassium is directed out of the cell, while the concentration gradient for sodium is directed into the cell.
It is important to understand equilibrium potentials in order to understand why the membrane potential is where it is in any circumstance.
Resting potential revisited[]
The resting potential of a cell can be most thoroughly understood by thinking of it in terms of equilibrium potentials. In the example diagram above, the model cell was given only one permant ion (potassium). In this case, the resting potential of this cell would be the same as the equilibrium potential for potassium.
However, a real cell is more complicated, having permeabilities to many ions. Each of these permeant ions will contribute something to the resting potential. To understand better, consider a cell with only two permeant ions, potassium and sodium. Consider a case where these two ions have equal concentration gradients directed in opposite directions (actually close to the actual case). Consider further that the membrane permeabilies to both ions are equal. K+ leaving the cell will tend to drag the membrane potential toward EK. Na+ entering the cell will tend to drag the membrane potential toward ENa. Given the condition that the permeabilities to both ions are the same, the membrane potential will, at the end of the Na/K tug-of-war, end up halfway between ENa and EK. ENa tends to be around +60 mV, while EK tends to be around −80 mV. So the two equilibrium potentials are 140 mV apart. Half way between them will thus be ENa: 70 mV from either, or −10 mV. The membrane under the stipulated conditions will rest at −10 mV. Note that even though the membrane potential at −10 mV is stable, it is not an equilibrium condition because neither of the contributing ions are in equilibrium. This membrane potential is supported by continual K efflux and Na influx.
Closer to the actual case for a real resting potential is that the permeability of Na is not equal to the K permeability, but rather is about 5% of the K permeability. Thus the membrane potential will not be right at EK, but rather depolarized from EK by an amount of approximately 5% of the 140 mV difference between EK and ENa. Thus, the cell's resting potential will be about −73 mV (again, not in equilibrium, but stable).
All other values of membrane potential[]
From the viewpoint of biophysics, there is nothing particularly special about the resting membrane potential. It is merely the membrane potential that results from the membrane permeabilities that predominate when the cell is resting. At any given moment, there are two factors for an ion that determine how much influence that ion will have over the membrane potential of a cell.
- That ion's "driving force" and,
- That ion's permeability
Intuitively, this is easy to understand. If the driving force is high, then the ion is being "pushed" across the membrane hard (more correctly stated: it is diffusing in one direction faster than the other). If the permeability is high, it will be easier for the ion to diffuse across the membrane. But what are 'driving force' and 'permeability'?
- Driving force: the driving force is the net electrical force available to move that ion across the membrane. It is calculated as the difference between the voltage that the ion "wants" to be at (its equilibrium potential) and the actual membrane potential (Em). So formally, the driving force for an ion = Em - Eion
- For example, at our earlier calculated resting potential of −73 mV, the driving force on potassium is 7 mV ((−73 mV) − (−80 mV) = 7 mV. The driving force on sodium would be (−73 mV) − (60 mV) = −133 mV.
- Permeability: is simply a measure of how easily an ion can cross the membrane.
So in a resting membrane, while the driving force for potassium is low, its permeability is very high. Sodium has a huge driving force, but almost no resting permeability.
However, consider another case—the peak of the action potential. Here permeability to Na is high and K permeability is relatively low. Thus the membrane moves to near ENa and far from EK.
The more ions are permeant, the more complicated it becomes to predict the membrane potential. However, this can be done using the Goldman-Hodgkin-Katz equation. By simply plugging in the concentration gradients and the permeabilities of the ions at any instant in time, one can determine the membrane potential at that moment. What the GHK equations says, basically, is that at any time, the value of the membrane potential will be a weighted average of the equilibrium potentials of all permeant ions. The "weighting" is the ions relative permeability across the membrane.
There is, really, very little else one needs to know in order to understand a cell's membrane potential under any condition.
Effects and implications[]
While cells expend energy to transport ions and establish a transmembrane potential, they use this potential in turn to transport other ions and metabolites such as sugar. The transmembrane potential of the mitochondria drives the production of ATP, which is the common currency of biological energy.
Cells may draw on the energy they store in the resting potential to drive action potentials or other forms of excitation. These changes in the membrane potential enable communication with other cells (as with action potentials) or initiate changes inside the cell, which happens in an egg when it is fertilized by a sperm.
In neuronal cells, an action potential begins with a rush of sodium ions into the cell through sodium channels, resulting in depolarization, while recovery involves an outward rush of potassium through potassium channels. Both these fluxes occur by passive diffusion.
See also[]
- Action potential
- electrochemical potential
- membrane biophysics
- Goldman Equation
- Signal (biology)
References[]
- Alberts et al. Molecular Biology of the Cell. Garland Publishing; 4th Bk&Cdr edition (March, 2002). ISBN 0815332181. Undergraduate level.
- Guyton, Arthur C., John E. Hall. Textbook of medical physiology. W.B. Saunders Company; 10th edition (August 15, 2000). ISBN 072168677X. Undergraduate level.
- Hille, B. Ionic Channel of Excitable Membranes Sinauer Associates, Sunderland, MA, USA; 1st Edition, 1984. ISBN 0-87893-322-0
- Nicholls, J.G., Martin, A.R. and Wallace, B.G. From Neuron to Brain Sinauer Associates, Inc. Sunderland, MA, USA 3rd Edition, 1992. ISBN 0-87893-580-0
- Ove-Sten Knudsen. Biological Membranes: Theory of Transport, Potentials and Electric Impulses. Cambridge University Press (September 26, 2002). ISBN 0521810183. Graduate level.
de:Membranpotenzial is:Hvíldarspenna
| This page uses Creative Commons Licensed content from Wikipedia (view authors). |